4 Essential Quantum numbers Chemistry

Atomic orbitals are regions of space around the nucleus where electrons are likely present. Each atomic orbital is characterized by a set of quantum numbers that describe its size, shape, and orientation. Quantum numbers are a set of four parameters that describe the unique quantum state of an electron in an atom. They provide essential information about the electron’s energy, position, and spin within an atom. These were introduced as a part of the quantum mechanical model of the atom, which replaced the older Bohr model.

Quantum Numbers

Quantum numbers are fundamental in understanding the electronic structure of atoms and predicting their chemical behavior. They help determine the arrangement of electrons in atomic orbitals, which in turn influences an element’s chemical properties, reactivity, and bonding behavior.

The four quantum numbers used to describe atomic orbitals are the principal quantum number (n), the azimuthal quantum number (l), the magnetic quantum number (m_l), and the spin quantum number (m_s). Here’s a brief overview of how these numbers relate to atomic orbitals:

Principal Quantum Number (n):

1. Denoted by the symbol ‘n.’
2. Determines the main energy level or shell in which an electron resides.
3. It can take on positive integer values (1, 2, 3, …) with each value representing a different energy level.
4. As ‘n’ increases, the energy level and average distance of the electron from the nucleus increase.
5. Number of Orbital in shell = n²
6. Number of electron in shell = 2n²
7. It tells us about radius of the shell.

As the Principal Quantum number (n) value increases, radius of the shell increases.

Ex: Calculate Radius r₁ , r₂ and r3 for hydrogen atom for n=1, n=2 & n=3.

8. It also gives information about energy of the shell.

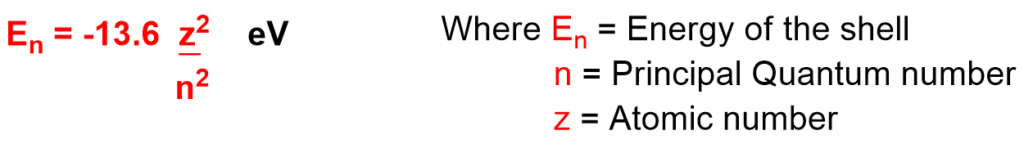
As the Principal Quantum number (n) value increases, energy of the shell decreases.

Ex: Calculate E₁ and E₂ for hydrogen atom for n=1 & n=2.

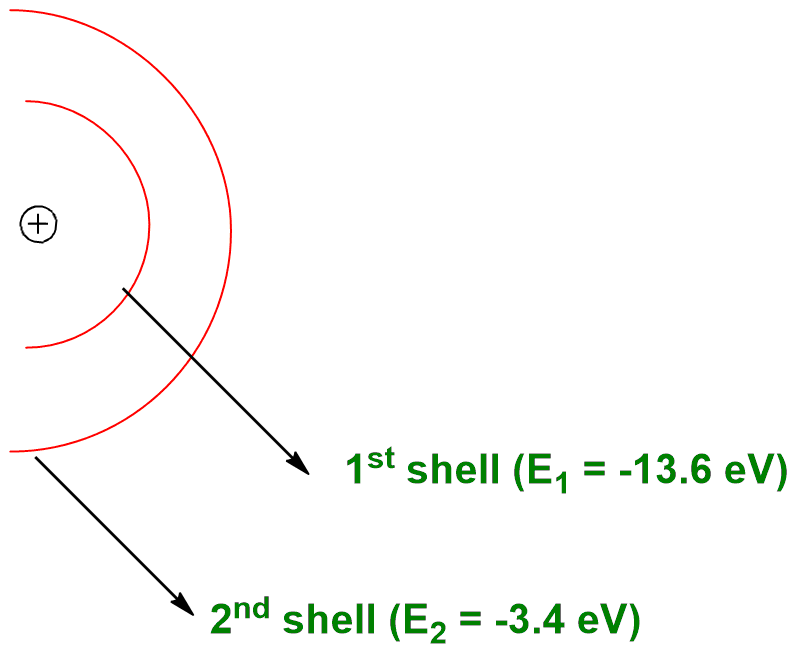
When n = 1, first shell has energy E₁ = -13.6 eV & n = 2. second shell has energy E₂ = -3.4 eV.

From this example we can conclude that as electron goes away from the nucleus. energy increases but stability decreases.

Azimuthal quantum number (l):

• Denoted by the symbol ‘l.’
• Also known as the Angular Momentum Quantum Number.
• Describes the shape or type of orbital an electron occupies within a given energy level.
• The possible values of ‘l’ range from 0 to (n-1).
• For ‘l’ values:
  • 0 corresponds to an s orbital (spherical)
  • 1 corresponds to a p orbital (dumbbell-shaped)
  • 2 corresponds to a d orbital (complex shapes)
  • 3 corresponds to an f orbital (more complex shapes)
• l value cannot be greater or equal to principal quantum number (n).
• Number of Orbital in subshell = 2l + 1
• Number of electron in subshell = 2(2l + 1)

Magnetic Quantum Number (m_l):

• Denoted by the symbol ‘m_l.’
• Specifies the orientation of the orbital in space.
• The possible values of ‘m_l‘ range from -l to +l, including zero.
  1. For s – subshell, l = 0, m_l = 0
  2. For p – subshell, l = 1, m_l = -1, 0, +1
  3. For d – subshell, l = 2, m_l = -2, -1, 0, +1, +2
  4. For f – subshell, l = 3, m_l = -3, -2, -1, 0, +1, +2, +3
• It determines how an orbital aligns itself in three-dimensional space.
• m_l values can never be greater than “l”.

Spin Quantum Number (m_s):

• Denoted by the symbol ‘m_s.’
• Describes the intrinsic spin of an electron.

• Electrons can have one of two possible values for their spin: +1/2 (clockwise) or -1/2 (anticlockwise).

• This quantum number is important because it helps distinguish between two electrons in the same orbital (Pauli Exclusion Principle).

To know more about the 4 quantum numbers refer the video.